Matric Notes Physics Notes Topic Scalar and Vectors

If you you want to view notes of Physics 9th Click Here.

If you you want to view notes of Physics 10th Click Here.

SCALAR

"Scalar quantity are those physical quantity which are completely specified by their magnitude express with suitable unit. They do not require any mention of the direction for complete their specification is called scalar quantity."

OR

" Scalar quantity are those physical quantity which require magnitude , express with suitable unit only is called scalar quantity."

CHARACTERISTICS OF SCALAR QUANTITY

1, Scalar quantity can be added, subtracted, multiplied, divided according to the ordinary algebraic rule.

2, Two scalars are equal if they have same unit.

REPRESENTATION

It can be represented by the numbers with decimals. (positive negative)

EXAMPLE

Mass, Distance, Temperature, volume, speed e.t.c.

VECTOR

"VECTOR quantity are those physical quantity which do not require only their magnitude express with suitable unit. But they also require a particular direction for complete their specification is called vector quantity."

OR

" vector quantity are those physical quantity which require magnitude , express with suitable unit as well as proper direction is called vector quantity."

CHARACTERISTICS OF VECTOR QUANTITY

1, vector quantity can not be added, subtracted, multiplied, divided according to the ordinary algebraic rule.

2, It can be added, subtracted, multiplied, divided according to the some special rules like head and tail rule, Graphical method e.t.c. 

3, vector always treats as positive.

REPRESENTATION

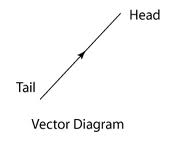
It can be represented by an arrow with headline. The length of an arrow represents its magnitude and the headline represents the direction of the vector(figure 1.1)

------------------------------------->

(figure 1.1)

EXAMPLE

Weight, Displacement, Velocity, Acceleraton, Torque, Momentum e.t.c.

ADDITION OF A VECTOR

"The process of combining of two or more vector to produce a signal vector having the combining effect of all the vector is called the resultant of the vector and this process is known as the addition of a vector".

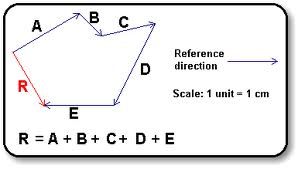
HEAD AND TAIL RULE

Suppose we have two vector A and B having the different magnitude and direction.

1, First of all chose a suitable scale and representation of all the vector have been drawn on the paper.

2, Put all the vector for finding the resultant of given vector such that the head of the first vector join the tail of the second vector.

3, Now join the tail of the first vector with tail of the second vector such that it join the two vector with head to head and tail to tail by another.

4, The new vector R will be the resultant of the given vector.

5, It can be measured by the Dee or any suitable mean. This method is called the head and tail or tip to tail rule.

RESOLUTION OF A VECTOR

"The process of splitting up of a signal vector into two or more vector is called the resolution of a vector"

OR

"The process of splitting up of a signal vector into its components is called the resolution of a vector"

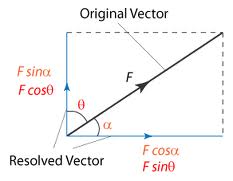
RECTANGULAR COMPONENTS

A vector which is not along x-axis or y-axis it can be resolved into infinite number, but generally a vector can be resolved into its components at a right angle to each other

MATHEMATICALLY PROVED

suppose a vector F is denoted by a line AB which makes an angle @ with horizontal surface OX. From a point A draw perpendicular to the horizontal surface OX.

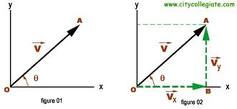
The line AB represents its vertical component and it is denoted by Fy. The line OB represents its horizontal component and it is denoted by Fx. Now in the triangle AOB

Sin@= AB/OA {sin@= Perpendicular/Hypotenuse}

or sin@= Vy/V

or Vy= V sin@

Similarly

Cos@= OB/OA {sin@= Base/Hypotenuse}

or Cos@= Fx/F

or Vx= V Cos@

For the triangle

Tan@= AB/OB {Tan@= per/hyp)

or Tan@= Vy/Vx

or @=Tan-1 =Vy/Vx

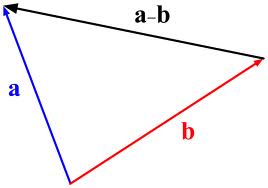
SUBTRACTION OF A VECTOR

"It is defined as the Addition of A to the negative of a B is called the subtraction of a vector (A-B)"